Don Steward’s blog ‘Median’: a source of high-quality teaching and practice materials
This blogpost was written by Dr Tom Francome. Tom’s work connects research, scholarship, and practice. He also leads Loughborough’s mathematics PGCE. His recent research focuses on practising mathematics and developing both mathematics and mathematicians through curriculum. There are links to learn more about Tom’s work, and the subject of this post, at the bottom of the blogpost. Edited by Dr Bethany Woollacott.
Don Steward’s median blog (https://donsteward.blogspot.com – linked at the end of this blogpost) is an invaluable repository of mathematics teaching resources. Don’s notes are being published for anyone who would like to go deeper. In this blogpost, Tom Francome remembers Don Steward and pays tribute to his work as a mathematics educator and task designer.
Introduction
Don Steward (1950-2020) inspired countless teachers and learners in his fruitful career as a mathematics educator and resource designer. He worked as a mathematics teacher and advisor for 47 years in Shropshire, UK, and led professional development across the country. Don was also an expert task designer, sharing his ideas through his advisory work, his company (MEDIAN), and later his popular blogsite (Median – linked at end) where he freely shared his resources for teaching mathematics.
Don’s hugely popular blog, where he’s published his delightful ‘tasks with depth’ since 2007, has been a source of reliably high-quality inspiration with millions of views from across the globe. The tasks are mainly aimed at learners in the 11-16 age range and are easily searched by topic or by clicking the links on the right-hand side of the page. Don designed his resources to support teachers in delivering engaging and thought-provoking mathematics lessons. His resources help develop problem-solving skills through carefully curated mathematical tasks that encourage deeper thinking.
The magic of Don’s mathematics teaching resources
Don’s teaching materials were often crafted with hidden depths, but one of his greatest talents was making these tasks feel approachable. To both pupils and teachers, they appeared like any regular exercise; however, while students could engage with them like simple exercises, they would often find themselves noticing and discussing interesting patterns and ideas along the way. Over time, and with a bit of exploration, teachers could uncover these deeper aspects, leading to richer discussions and greater insights from their students.
I was fortunate to know Don, having first met him when I was a newly-qualified teacher in 2003 during a local authority training day. Somehow that day he managed to share hundreds of ideas from his ‘Median’ resources, alongside insightful ways of bringing out the richness of the mathematics and getting children noticing and generalising relationships. He profoundly influenced my teaching from then on. I got to know him better through professional development meetings, conferences and conversations about developing teaching, particularly on the mathematics PGCE where he led brilliant sessions that were different every time. He was always generous and supportive of me and this generosity extended to the wider community as he was always willing to give up his time to talk or provide materials or ideas for others. Sadly, on 3rd May 2020 Don died of Covid-19.
The last time I saw Don, we spent a day planning our joint ATM1 conference session just before the first lockdown. As always, he was generous, interested and excitable, and I was looking forward to working with him much more in the future. He had offered to support us in developing the LUMEN Curriculum (Loughborough University’s free, research-informed secondary mathematics curriculum, linked at the end of this blogpost), and whilst some of his ideas are adapted there, their main home is his blog.
I said in Mathematics Teaching:
“Anyone wanting to know how to create lovely maths tasks might like to know Don’s secret. He would sit with a coffee and play. Just play about with the mathematics until something lovely happened. And that is what his tasks offer pupils – an opportunity to play with mathematics and for something worth talking about to be revealed.”
~ Tom Francome (Francome et al., 20202, p.42)
However, that might not offer enough insight into the thinking. Mathematics teachers like it when they get to see people’s working. So, when Don’s paper notes were passed on to us, we thought others might enjoy seeing how his ideas evolved and what influenced his thinking. Don’s family gave us permission to digitise the materials to be shared on his blog. Don’s notes on tasks for teaching mathematics were stored in over 140 ring-binders. The tasks are mainly aimed at learners aged 11-16 and the notes include early versions of tasks on his blog, some of the paper-based Median resources, and notes, workings, and ideas in development.
It took quite a while to do the scanning – consisting of over 22,000 pages and presented somewhat idiosyncratically. While Don’s blog is easily navigated to quickly find something you need, these notes are better suited to more leisurely browsing. Don’s notes are now freely available on his site (link at end).

Some favourite lessons from Don
Here I offer some examples of the kinds of material available in Don’s notes that might pique your interest. There are lots of Don’s ideas I might have drawn on here by way of example – I’m particularly fond of his classic median worksheets.
‘2 congruent halves’ is perhaps my favourite ever worksheet and I was excited to find an original hand drawn version within his notes. [Shape bits, p.119]
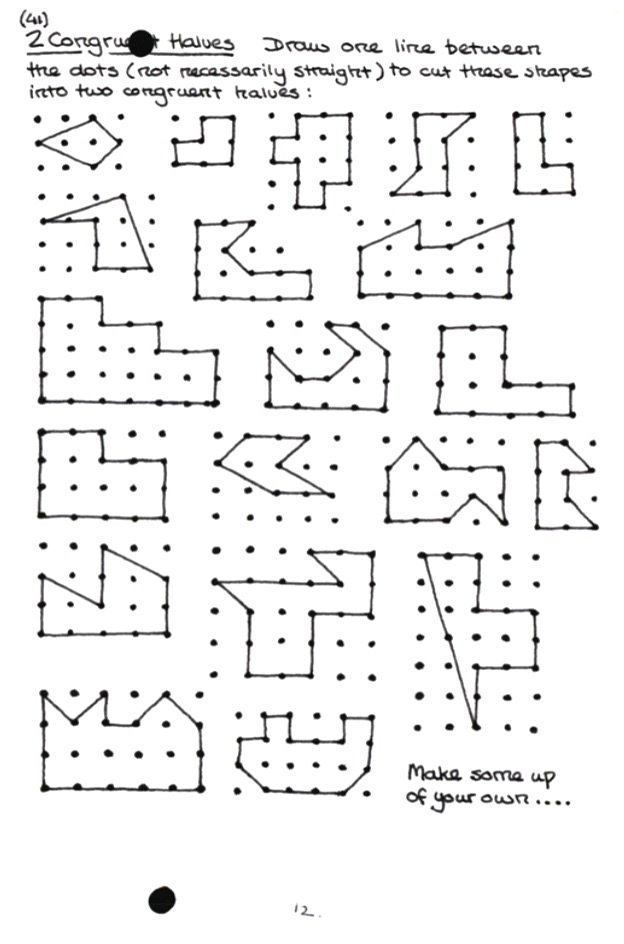
He developed this task over time and a number of variations can be found on his site. For example, if you want something a bit more open:
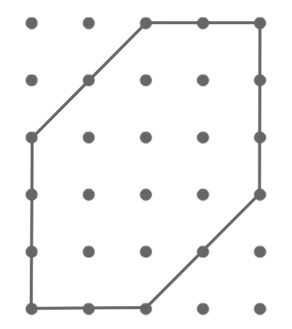
How many ways can you dissect this shape into two congruent halves?
For a while, Don and I were both working on the idea that school geometry could be helpfully introduced by working where possible within the structure of a grid. I was very excited to see some notes he’d made on a task he developed and gave to my PGCE students. I thought I’d lost the only paper copy! This task exploited the grid structure and askes learners to complete shapes to make a certain fraction or area of a rectangle. You can see part of the task below.
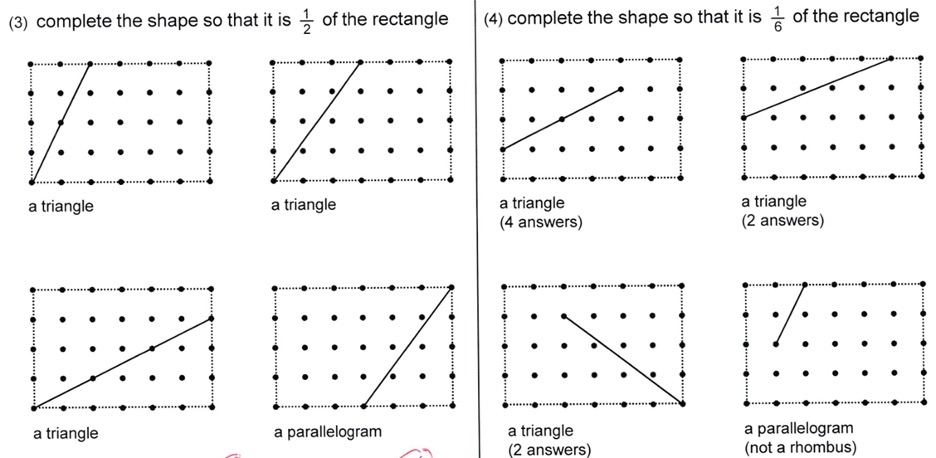
His notes also provide some insight into the level of care he put into developing tasks as he worked on and reordered problems to provide the best mathematical experience.
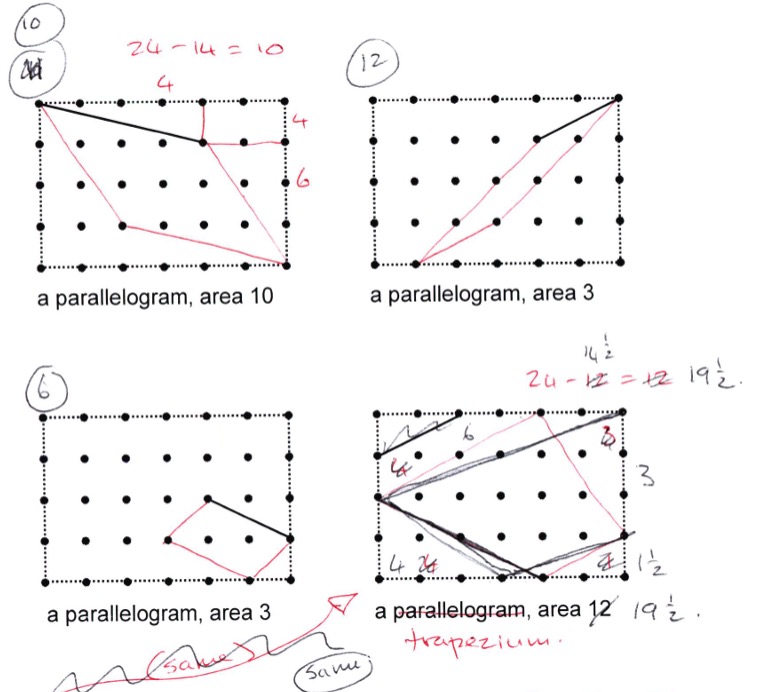
I expanded on the idea of exploiting the grid structure in the Mathematical Association’s Journal, ‘Mathematics in School’’s tribute to Don3 and also within, in particular, the Length and Area module of the LUMEN Curriculum (you can find links to these, and others, at the end).
Don’s Legacy
Don would have described himself as a ‘collector’ of resources, but he was also an innovator – constantly tinkering, adapting, extending and polishing tasks – adding his own sparkle to make the tasks shine. He never considered any of the tasks to be ‘his’, but wanted his work to remain freely available to help teachers and learners. He hoped people who use his resources might donate to charities supporting education in Africa, so please consider doing so if you are able: https://www.justgiving.com/fundraising/jessesteward
On Don’s website, there are many tasks that develop mathematical thinking in interesting and thought-provoking ways and I would encourage anyone who hasn’t seen it to spend some time exploring his blog. If you’d like to know more, we hope you will find something of interest within Don’s notes. If you are interested in task design, you may like to see how early versions of tasks (such as two congruent halves above) evolved. You might also be able to see where some of the inspiration came from for Don’s more polished ideas. As Dave Hewitt noted,
“[Don] was always interested in what others were doing but also saw little gems within those things that he adapted and developed in ways which suited his way of working. This mix of being open to new ideas, whilst having a strong sense of the way in which he wanted to teach mathematics, was very powerful.”
~ Dave Hewitt (Francome et al., 20202, pp. 42-43)
Although he was an expert task designer, he was relentlessly modest and was always eager to credit others for inspiring him.
Losing Don has been a great loss to me personally and to the profession, but thankfully the huge contribution he has made will live on to inspire future teachers and mathematicians.
Note: This work was made possible thanks to generous support from Loughborough University’s Centre for Mathematical Cognition funded by Research England.
Don’s blog contains all the polished tasks, resources and ideas for teaching mathematics to 10 to 16 year-old students. They are for teachers “who like tasks with some depth, novelty and a focus on generalising relationships and on transformation”. These resources can be used, reproduced, and modified, for non-commercial purposes by teachers and learners. If you want tasks on a particular area of mathematics, then look at the topic menu on the right hand side. Click to bring up all posts on that topic.
As noted above, we had permission to digitise Don’s paper notes and these were recently uploaded to his site. They can be accessed by clicking one of the eight folders at the bottom of the page. This takes you to Google Drive where you can view the files.
Don wanted his work to support the teaching of mathematics so please feel free to use it in your teaching. This material (and other material on Don’s blog) may be reproduced, and modified, for non-commercial purposes, under the Creative Commons license CC BY-NC-SA 4.0 detailed at https://creativecommons.org/licenses/by-nc-sa/4.0/ – all other rights reserved by Don’s family. If you recognise any third-party materials that you hold the copyright of then please email: Dme@lboro.ac.uk
References
1. Association of Teachers of Mathematics: https://atm.org.uk/
2. Francome, T., Sugarman, S., Hewitt, D., Pitt, A., and Charles, G. (2020) ‘Remembering Don Steward’, Mathematics Teaching, 272, pp. 42-43. https://www.m-a.org.uk/MathematicsinSchool/50.3/16/index.html
3. Francome, T. (2021). ‘Transforming the way you teach quadrilateral properties’. Mathematics in School, 50(3), pp. 12-14. https://doi.org/10.17605/OSF.IO/Z5JV7
Further reading
Shape
The files referenced above can be found in 8 – ‘shape bits’ https://drive.google.com/drive/folders/1TGCfIFww4skIdAdtQz0sW4BWo5jSw5SZ
You can find Two Congruent Halves on p. 161, and Fraction Rectangles on p. 223.
The newer versions of exploring ideas of congruency can be found here: https://donsteward.blogspot.com/search/label/congruent%20parts
Building on Don’s work of using grid structures to help with learning mathematics, I suggest ways grids can be exploited here in the Mathematical Association’s Journal, Mathematics in School’s tribute to Don: Francome, T. (2021). ‘Transforming the way you teach quadrilateral properties’, Mathematics in School, 50(3), pp. 12-14. https://doi.org/10.17605/OSF.IO/Z5JV7
Additional applications of Don’s work:
We used many of Don’s ideas within the LUMEN Curriculum, in particular, see the Length and Area module of the LUMEN Curriculum: https://www.lboro.ac.uk/services/lumen/curriculum where grids are used very intentionally. More of Don’s impressive thinking on this topic can be found on his blog here: https://donsteward.blogspot.com/search/label/angles%20on%20a%20grid.
Anne Watson has also expanded on some of Don’s ideas in more depth here: http://www.pmtheta.com/dose-of-don.html
Centre for Mathematical Cognition
We write mostly about mathematics education, numerical cognition and general academic life. Our centre’s research is wide-ranging, so there is something for everyone: teachers, researchers and general interest. This blog is managed by Dr Bethany Woollacott, a research associate at the CMC, who edits and typesets all posts. Please email b.woollacott@lboro.ac.uk if you have any feedback or if you would like information about being a guest contributor. We hope you enjoy our blog!